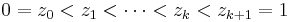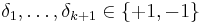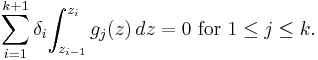Hobby–Rice theorem

In mathematics, and in particular the necklace splitting problem, the Hobby–Rice theorem is a result that is useful in establishing the existence of certain solutions. It was proved in 1965 by Charles R. Hobby and John R. Rice;[1] a simplified proof was given in 1976 by A. Pinkus.[2]

The theorem

If

g_1,\ldots,g_k:[0,1]\longrightarrow\Bbb{R} \,

are given continuously integrable functions then there exist

0=z_0 < z_1 < \cdots < z_k < z_{k%2B1} = 1 \,

and

\delta_1,\ldots,\delta_{k%2B1}\in\left\{%2B1,-1\right\}

such that

\sum_{i=1}^{k%2B1}\delta_i\!\int_{z_{i-1}}^{z_i} g_j(z)\,dz=0\text{ for }1\leq j\leq k.

The theorem was used by Noga Alon in the context of necklace splitting[3] in 1987.

References

  1. ^ Hobby, C. R.; Rice, J. R. (1965). "A moment problem in L1 approximation". Proceedings of the American Mathematical Society (American Mathematical Society) 16 (4): 665–670. doi:10.2307/2033900. JSTOR 2033900. 
  2. ^ Pinkus, Allan (1976). "A simple proof of the Hobby-Rice theorem". Proceedings of the American Mathematical Society (American Mathematical Society) 60 (1): 82–84. doi:10.2307/2041117. JSTOR 2041117. 
  3. ^ Alon, Noga (1987). "Splitting Necklaces". Advances in Mathematics 63: 247–253. doi:10.1016/0001-8708(87)90055-7.